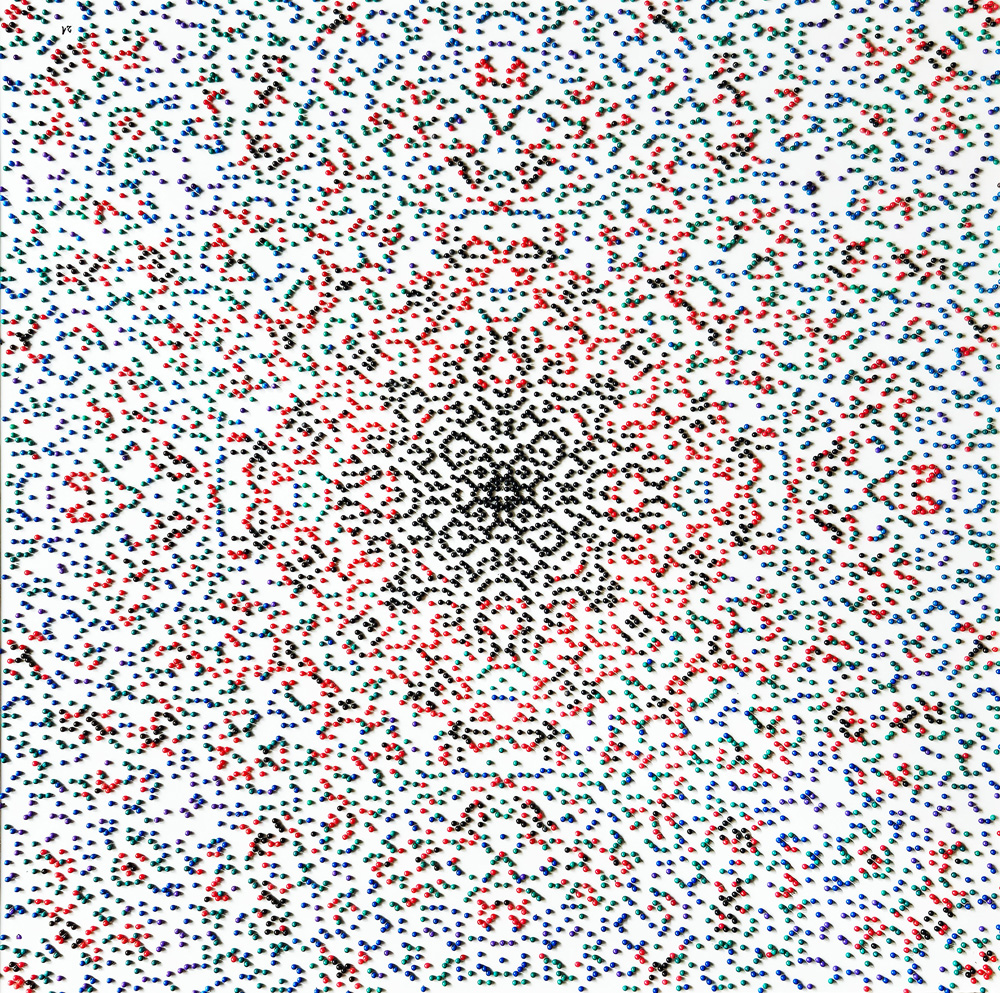
Gaussian and Eisenstein Primes

Until the middle of the 17th century, mathematics was largely confined to static questions of counting, measuring, and describing shapes. Then, independently, Gottfried Wilhelm Leibniz and Isaac Newton made perhaps the most significant advancement in the history of mathematics with the invention of differential and integral calculus. The introduction of infinitesimal calculus, as it was summarily called, enabled the mathematical handling of motion and change. With this technique, mathematicians began to study the motions of planets, the falling motion of bodies on Earth, the mechanisms of machines, the flow of fluids, the expansion of gases, physical forces, magnetism and electricity, flight, the growth of plants and animals, the spread of epidemics, changes in economic gains, and so on.

Moreover, mathematical methods began to be used to investigate mathematics itself. This, in turn, led to the recognition of a number system that encompassed even seemingly impossible entities such as negative square roots. Although the ancient Greeks knew how to handle negative numbers, it was not until the 18th century that negative numbers were recognized as real numbers. And it took even longer for it to be accepted that the square root of a negative number could be a real number. The resistance to these numbers is reflected in the term "imaginary number." Dealing with negative square roots became possible when the Swiss mathematician Leonhard Euler defined the square root of -1 as a constant "i," standing for imaginary (i = √-1). Then, the square root of any negative number -a is simply i√a, the product of the special number i and the square root of the positive number a.

The German mathematician Carl Friedrich Gauss developed from this the system of complex numbers of the form a + bi, where a and b are real numbers. Along with the rule that i^2 = -1, complex numbers in this system can be added, subtracted, multiplied, and divided according to the usual rules of algebra. By starting to apply complex numbers to functions, number theorists could find new number patterns leading to further mathematical insights even in the realm of natural numbers (see Riemann's hypothesis for understanding ordinary prime numbers).

The patterns depicted here represent prime numbers in the set of complex numbers, which differ from ordinary prime numbers. The different colors correspond to different types of prime numbers, such as those of the form a + bi, which may have an even component in the real components (a or b), but as complex numbers, are only divisible by 1 and themselves.