Stomachion

 

 

 

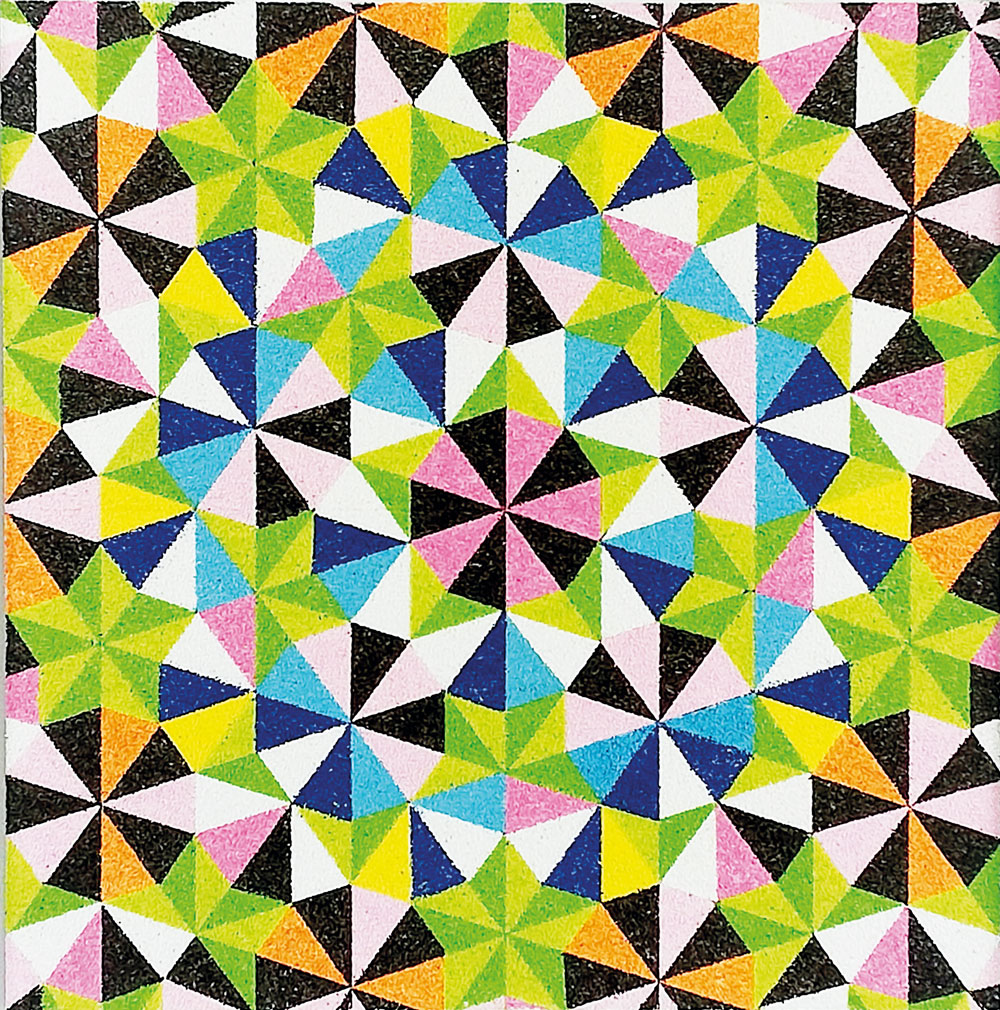Penrose Tiling

The tessellation of a surface with triangles, squares, or hexagons is called periodic because they can cover the surface seamlessly and without overlapping in all directions.



For decades, mathematicians were preoccupied with the question of whether certain tiles could lead to an infinite, non-periodic (aperiodic) tiling without gaps or overlaps, meaning that the pattern does not repeat itself even when shifted in any direction (left, right, up, down, and diagonally). Such a pattern would lack translational symmetry.

The possibility of covering the plane with an aperiodic tiling was first proven in 1966 by Robert Berger, who shortly afterward published an example using 20,426 different tiles. Subsequently, smaller sets of tiles for an aperiodic tiling were presented until physicist Roger Penrose (Nobel laureate in Physics 2020) reduced the number of tiles to two in 1973.

There are several versions of Penrose tiling, with the most famous one consisting of a thick and a thin rhombus. However, it is crucial to adhere to the rule that two rhombuses cannot be combined to form a parallelogram. These rhombuses have the same edge length but different internal angles. Their respective areas and their number in the tiling (inflation factor) are in the ratio of the Golden Ratio.
Penrose tiling with thick and thin rhombuses (colored sand on lightweight panel, 60 x 60 cm).

Atom model of an Al-Pd-Mn (aluminum-palladium-manganese) quasicrystal.

The aperiodic Penrose tiling foreshadowed the mathematical discovery of so-called quasicrystals, which were previously thought to be impossible. In a regular crystal (such as salt), the atoms or molecules are arranged in a periodic structure, similar to periodic tiling. Each unit cell is surrounded by cells forming an identical pattern. Accordingly, in regular crystals, only two-, three-, four-, and six-fold symmetries are possible. However, in 1982, Israeli physicist Daniel Shechtman discovered an aperiodic crystal structure during the structural analysis of an aluminum-manganese alloy, where, similar to aperiodic tiling, no lattice translations are possible. Each unit cell is surrounded by a different pattern. Shechtman was awarded the Nobel Prize in Chemistry in 2011 for this discovery. When a quasicrystal is cut accordingly, the cross-section essentially displays the pattern of Penrose tiling.